28 faszinierende Modelle bauen, die rechnen, zeichnen und messen
Die ersten Mitbau-Videos stehen auf meinem YouTube-Kanal bereit. Einige weitere werden in unregelmäßigen Abständen folgen. Der Link führt auf eine externe Seite mit ihren eigenen Datenschutzbestimmungen.
Zum xy-Schreiber gibt es auf der entsprechenden Seite unten eine .ftm-Datei für den fischertechnik-Designer.
| 13.10.2023 | Mathematikum Gießen | Tag des Rechnens |
| 15.4.2023 | Schulzentrum Mellendorf | Nordconvention |
| 29.01.2023 | KvG-Gymnasium Münster | Modellausstellung |
Mathematik: konkret, konstruktiv, kreativ.
28 Modelle zum Nachbauen und Experimentieren laden dich zu einer Reise durch die Welt der Mathematik ein. Durch die rechnenden, zeichnenden und messenden Apparate lernst du mathematische Kernkonzepte aus einer neuen, faszinierenden Perspektive kennen. Thematisch spannt das Buch einen weiten Bogen durch die Schulmathematik und darüber hinaus - von einfachen Instrumenten wie dem Zirkel über funktionsfähige Rechenmaschinen bis hin zu Apparaten, die Gleichungen lösen, Sinusfunktionen grafisch überlagern oder Zahlen faktorisieren.
Das Buch ist in einem motivierenden Fragen-und-Antworten-Stil geschrieben. Dadurch kannst du selbst bestimmen, ob du über das experimentelle Verständnis zu einem Thema hinausgehen möchtest und wie weit du die Theorie dahinter verstehen möchtest. Zu jedem Modell gibt es eine detaillierte, erprobte fotografische Bauanleitung.
Hier stelle ich nach und nach Zusatzinformationen zu den Modellen bereit (Varianten, Videos, Links, Ergänzungen, ...).
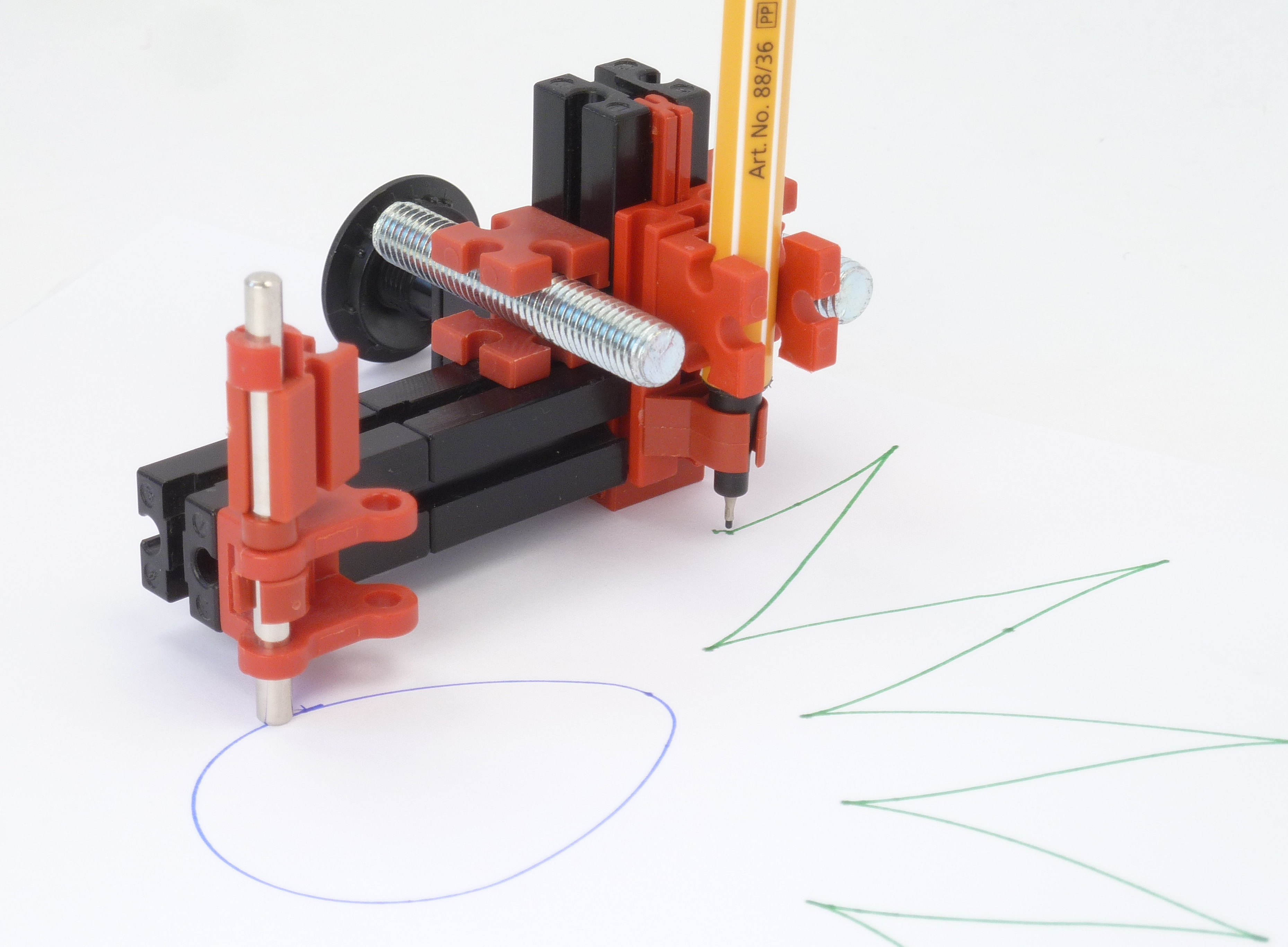
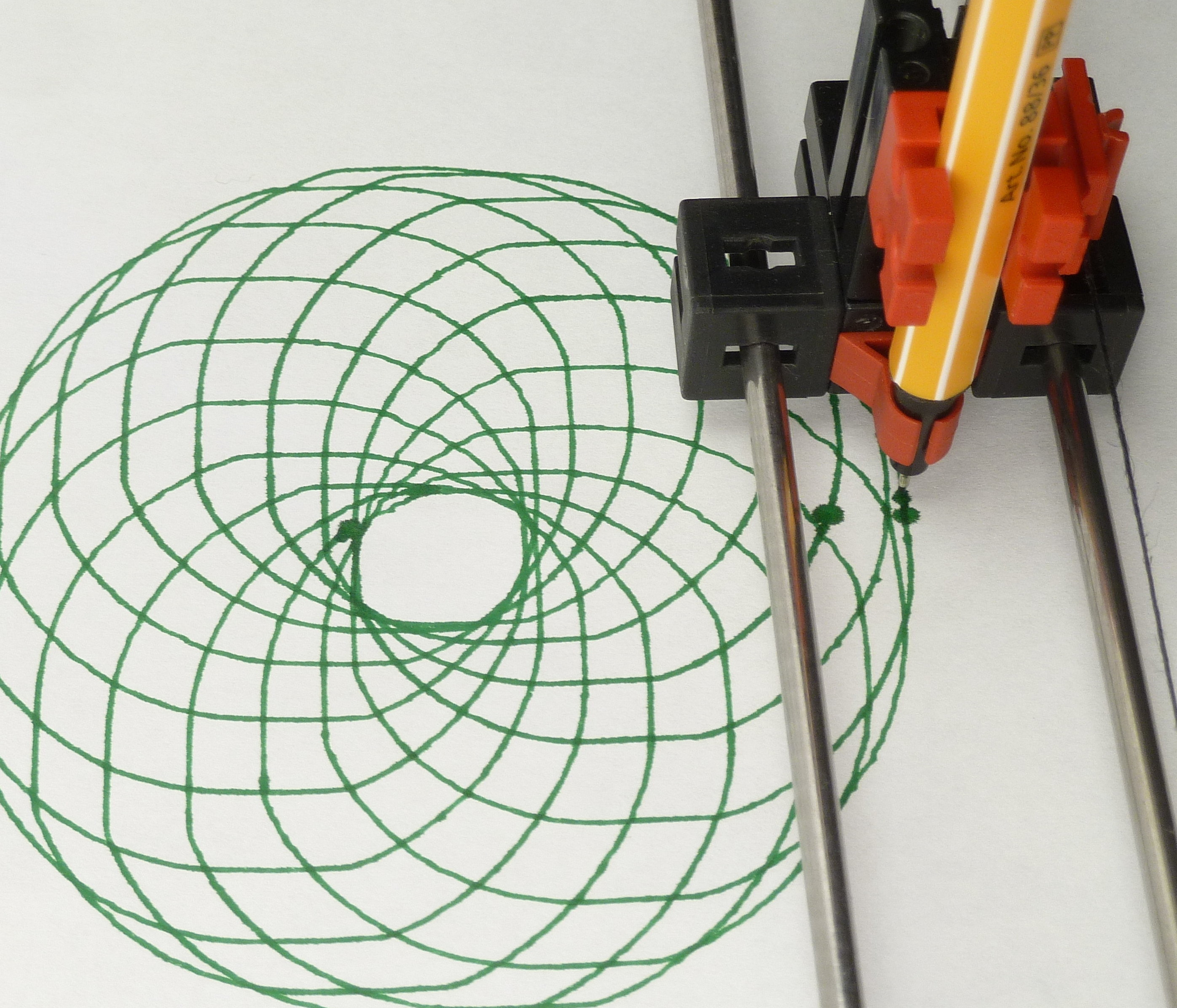
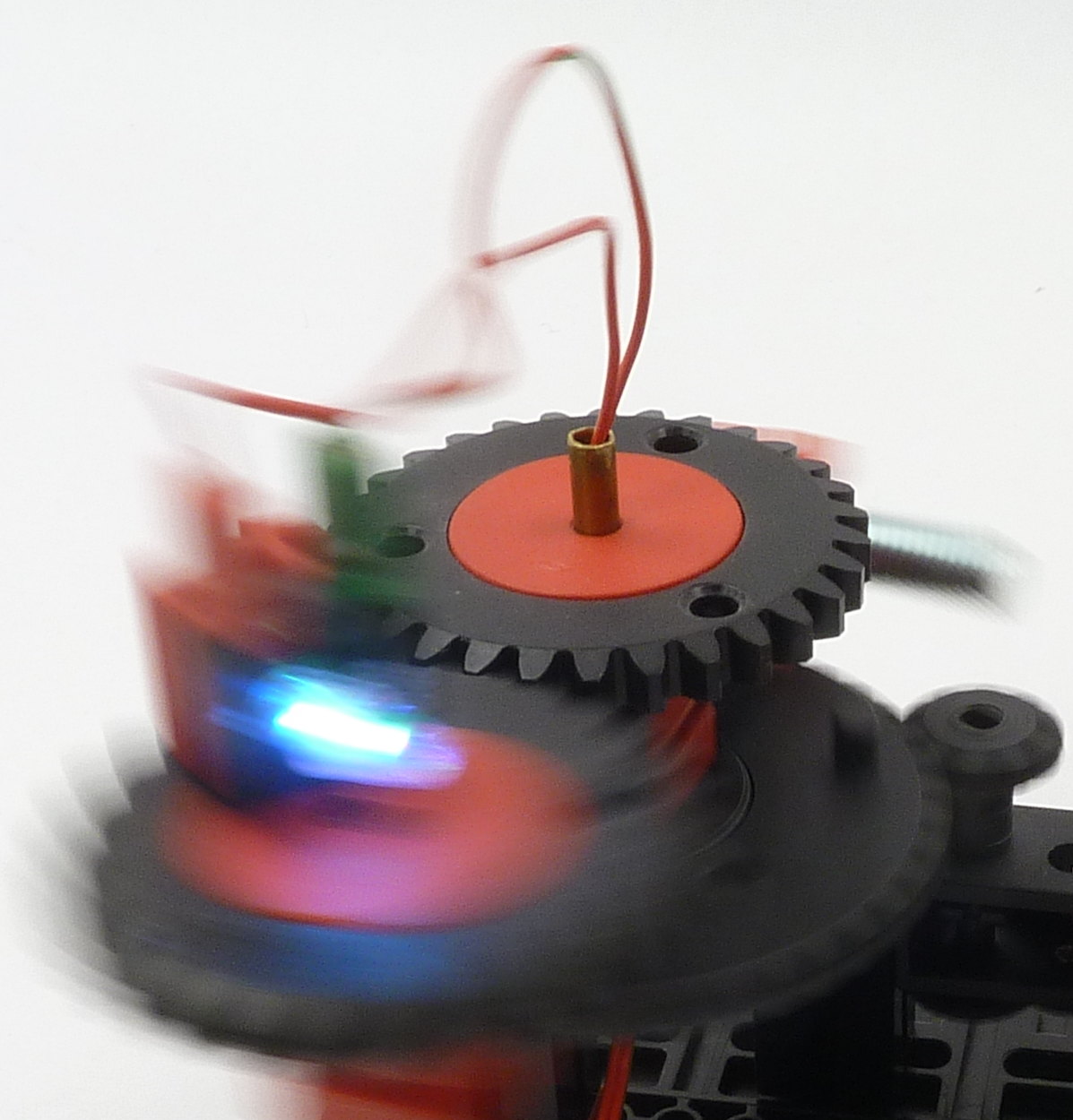
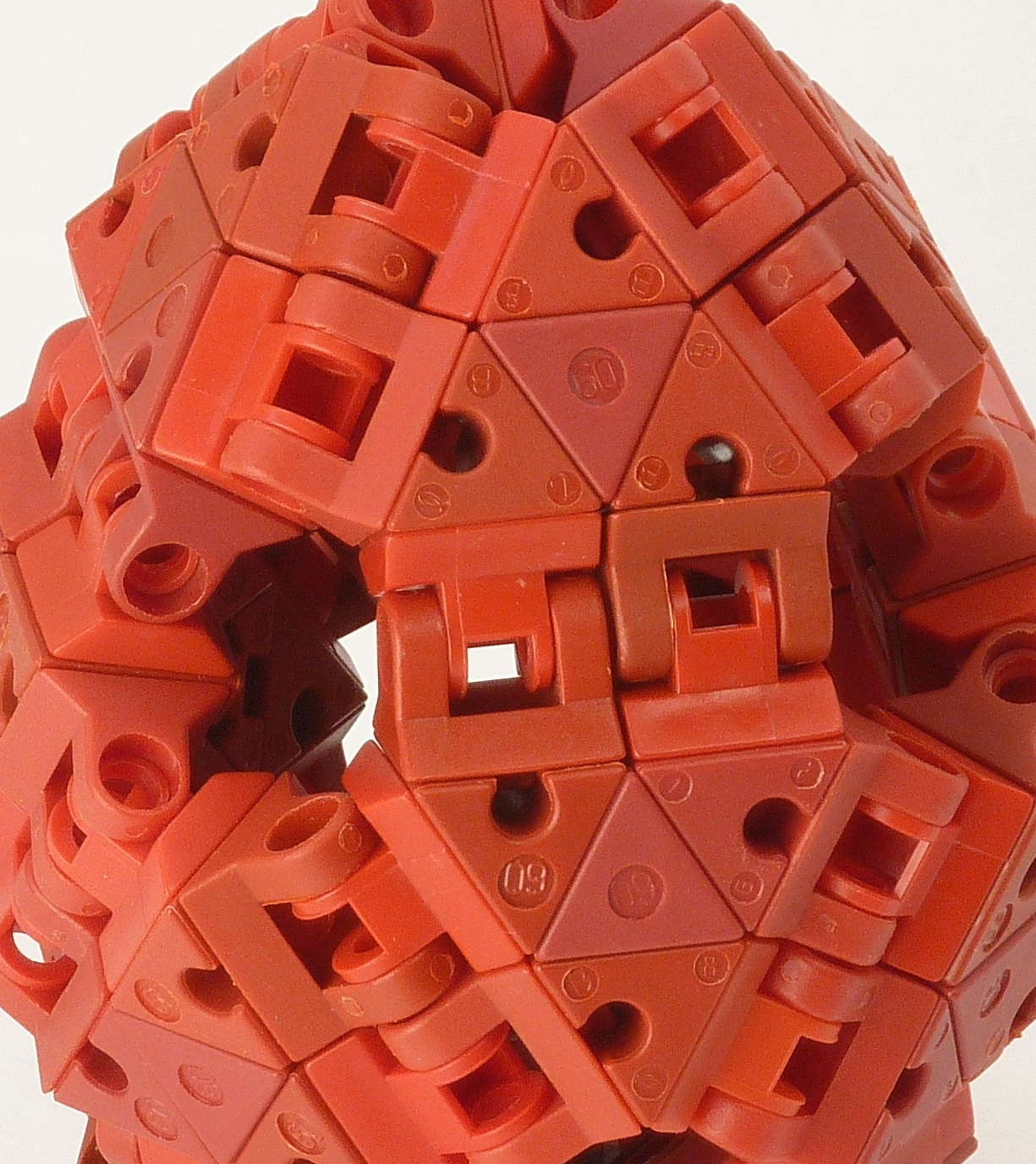
Dieses Buch ist für dich geeignet - ganz gleich, ob oder wie viel fischertechnik du besitzt. Was immer dir an Teilen fehlt, kannst du nach und nach bestellen.
fischerfriendsman liefert dir gerne, was du benötigst. Auch die wenigen Fremdteile (Klebeband, Laserdiode, Messingrohr, ...) kannst du bei ihm bekommen. Am flexibelsten geht das über die Excel-Liste auf seiner Seite zum Buch.
Der Sextant kostet so zum Beispiel mit bespielten Teilen nur 15 Euro. Damit dürfte er der günstigste voll funktionsfähige Sextant auf dem Markt sein.
Und mit 382 Teilen für etwas mehr als 40 Euro kannst du alle platonischen und viele andere spannende Körper bauen. Tagelanger Bauspass ist garantiert. Zum Vergleich: Das entsprechende Set „Die Platonischen Körper“ eines in der Mathematik sehr beliebten Herstellers kostet ca. 75 Euro.
Meine Originallisten im pdf-Format kannst du über den Button hier herunterladen.
Thomas Püttmann ist außerplanmäßiger Professor für Mathematik an der Ruhr-Universität Bochum. Die Modelle in diesem Buch hat er über viele Jahre in Workshops für Schülerinnen und Schüler und in universitären Seminaren erprobt und verbessert. Gemeinsam mit Dirk Fox hat er die Bücher Technikgeschichte mit fischertechnik und fischertechnik-Roboter mit Arduino geschrieben, die ebenfalls im dpunkt.verlag erschienen sind. Er ist verheiratet und hat vier Kinder.
Daniel Bachfeld schreibt in der Make: 6/2022:
Das Buch macht Spaß, selbst wenn man die Modelle nicht unbedingt nachbauen will.
Daniel Pietrzik schreibt auf Mediennerd.de
Die Anleitungen und Erklärungen sind verständlich, auch wenn man das Mathe-Wissen vorher noch nicht hatte. Ich kann euch dieses Buch mehr als empfehlem, schaut unbedingt selbst mal rein ... 9.4/10